267 ÷ 23 = ???

1. Start by putting the larger number (dividend) into a little “house” called the “vinculum” and the smaller number (divisor) outside of it

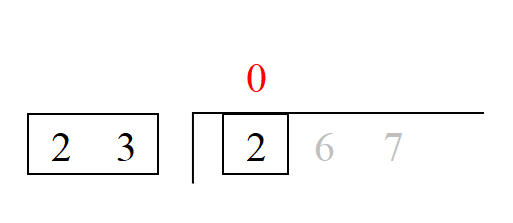
2. Look at the divisor and the first highest number of the dividend. How many times does the divisor go into the first number? 23 does not go into 2 at all since 23 > 2

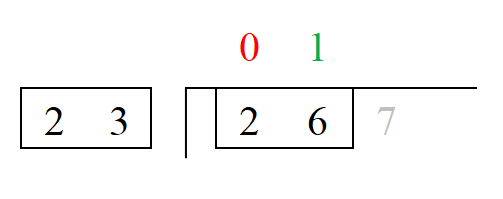
3. We need to include the second number of the dividend. That makes it 26. Since 23 < 26, it is divisible. 23 only goes into 26 1 time evenly so we write a 1 on top of the house in the 10s place.

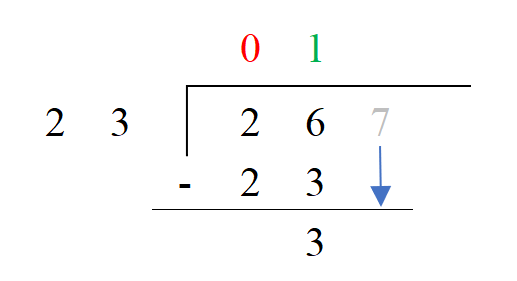
4. Subtract the divisor from the augmented dividend. In this case it is 26 – 23 = 3. We still need to deal with the 7 so we will drop it down.

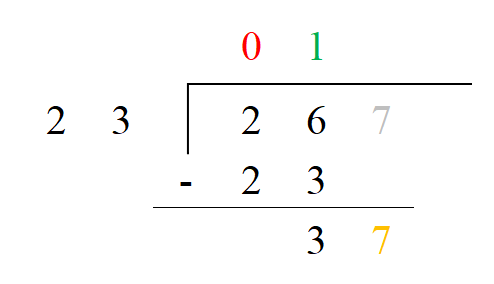
5. Look at the new value under the line and see how many times the divisor will go into that number. Since 23 < 37, it is divisible but it only goes into it one time. We write another 1 on top of the house in the ones place.

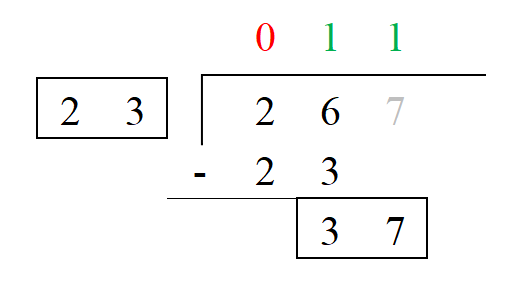
6.  Subtract the divisor value from 37. This gives us 14. Since 14 < 23, we cannot divide further

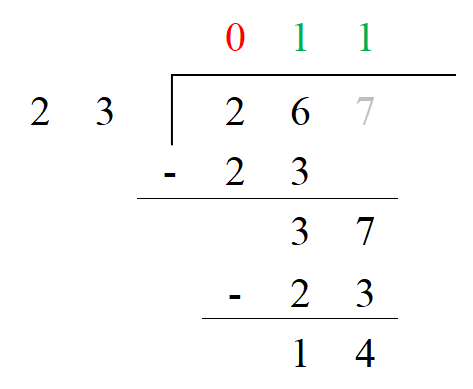
7.  The answer would be 11 with a remainder of 14 or 11 with 14/23 left over.

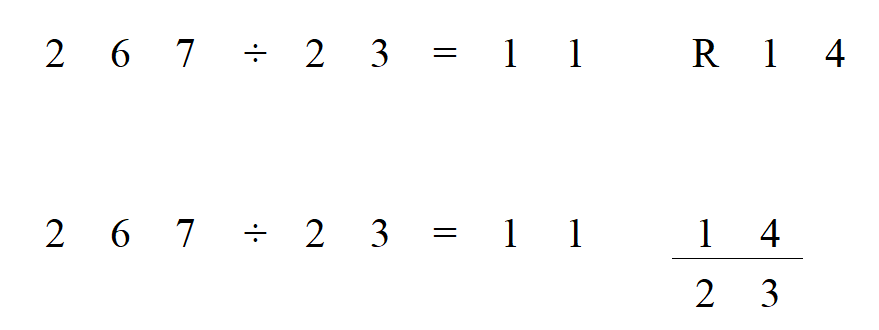
So 654 x 31 = 11 R 14